Patterns on the Genus-3 Klein Quartic
Abstract
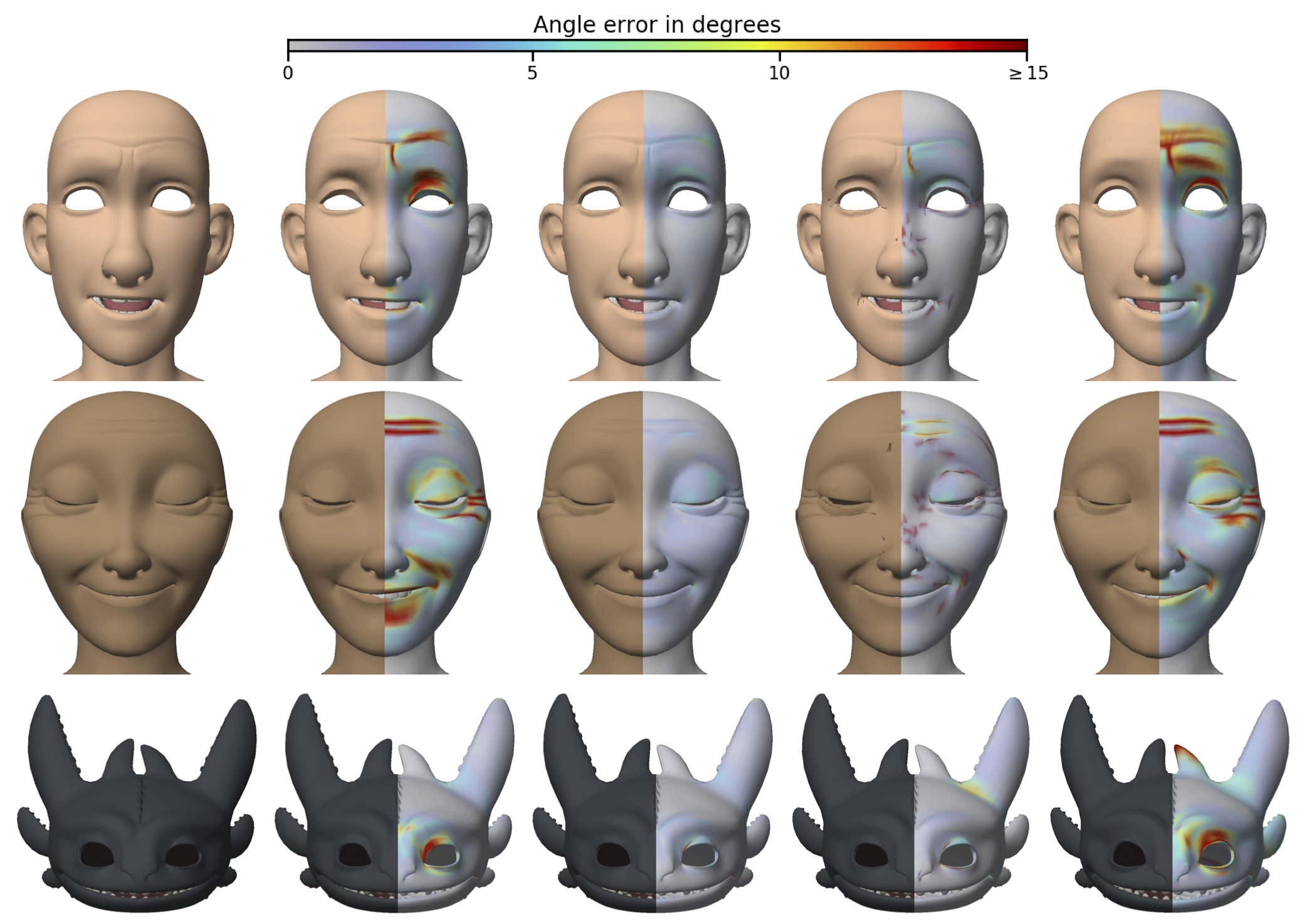
Projections of Klein's quartic surface of genus 3 into 3D space are used as canvases on which we present regular tessellations, Escher tilings, knot- and graph-embedding problems, Hamiltonian cycles, Petrie polygons and equatorial weaves derived from them. Many of the solutions found have also been realized as small physical models made on rapid-prototyping machines.
Citation
Carlo H. Séquin. "Patterns on the Genus-3 Klein Quartic". Bridges Conference, London, pages 245–254, July 2006.